Math
Sub Category
Potens - Potensopløftning - Hvad er en potens i matematik? - Lær hurtig hvordan man regner med potens? - Potensregneregler.
Videoen forklarer hurtigt og enkelt hvordan man regner med potens og rod i matematik.
Populært kaldet at sætte noget i anden, i tredje osv.
*HJEMMESIDER OM MATEMATIK* 😃 (Video med en kort præsentation af de enkelte sider her: https://www.youtube.com/watch?v=nHfq43oEgxg )
👉 https://mattip.dk/ - Gratis opgaver og kopiark.
👉 https://matematikbogen.dk/ - Print let opgaver om plus, minus, gange og dividerer gratis.
👉 https://www.gratisskole.dk/ - Masser af gratis skolerelevante opgaver.
👉 https://www.legoglektie.dk/ - Hjemmeside med skolematerialer til børn fra 2-13 år. Gratis at bruge for private.
👉https://www.matematikbanken.dk/side/kompendier/ - Blandet opgaver målrettet 9-10 klasse.
👉https://www.opgaveskyen.dk/ - Opgaver til mange af folkeskolens fag. Klar til print.
👉https://undervisningsforlob.dk/matematik - Matematikopgaver og små spil klar til print. Målgruppe 5-12 år.
👉https://www.printland.dk/sjov-med-tal.aspx - Print opgaver til de helt små børn (børnehave og tidlig indskoling).
*BUTIKKER*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=69642 - Online-butik hvor man kan købe *piktogrammer,* læringsspil, opgaver, tavler, legetøj og læringscirkler. *Stort udvalg*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=64474&htmlurl=https://www.laerlet.dk/butik/ - Online-butik med materialer der via leg og læring træner tal og bogstaver.
*APPS*
👉 *Reklamelink* - https://track.adtraction.com/t/t?a=1646486406&as=1772625203&t=2&tk=1 - *SUPER GOD matematikapp* til de mindste. Gratis prøveperiode.
*LEKTIEHJÆLP*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=95261 - *LEKTIEHJÆLP* både til folkeskole og gymnasie niveau - *Prøv gratis 👩🎓*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=76792 - BOOST dine matematikevner med et matematikforløb eller lektiehjælp.
*LÆRINGSCAMP*
👉https://www.dincamp.dk/camps/matematikcamp/ - Tag på camp i din ferie: Et sjovt fagligt boost i matematik (eller andre fag)
#Matematik
#Potens
#PotensRegneregler
#Potensopløftning
#PotensOgRoden
#UNIKSkole
#IAnden
#Regn
#RegnIAnden
#Lektier
#Lektiehjælp
Tælletræ - Hvad er det? Hvordan et tælletræ til at regne?
Få svaret og en forklaring her hurtigt og let :)
Lær hvordan man bruger et tælletræ til at hjælpe med at regne med sandsynlighed. Videoen forklarer brugen af tælletræ og arbejder med forståelse samt eksempler.
Folkeskolematematik
1. Klasse matematik
2. Klasse matematik
3. Klasse matematik
4. Klasse matematik
5. Klasse matematik
6. Klasse matematik
7. Klasse matematik
*HJEMMESIDER OM MATEMATIK* 😃 (Video med en kort præsentation af de enkelte sider her: https://www.youtube.com/watch?v=nHfq43oEgxg )
👉 https://mattip.dk/ - Gratis opgaver og kopiark.
👉 https://matematikbogen.dk/ - Print let opgaver om plus, minus, gange og dividerer gratis.
👉 https://www.gratisskole.dk/ - Masser af gratis skolerelevante opgaver.
👉 https://www.legoglektie.dk/ - Hjemmeside med skolematerialer til børn fra 2-13 år. Gratis at bruge for private.
👉https://www.matematikbanken.dk/side/kompendier/ - Blandet opgaver målrettet 9-10 klasse.
👉https://www.opgaveskyen.dk/ - Opgaver til mange af folkeskolens fag. Klar til print.
👉https://undervisningsforlob.dk/matematik - Matematikopgaver og små spil klar til print. Målgruppe 5-12 år.
👉https://www.printland.dk/sjov-med-tal.aspx - Print opgaver til de helt små børn (børnehave og tidlig indskoling).
*BUTIKKER*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=69642 - Online-butik hvor man kan købe *piktogrammer,* læringsspil, opgaver, tavler, legetøj og læringscirkler. *Stort udvalg*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=64474&htmlurl=https://www.laerlet.dk/butik/ - Online-butik med materialer der via leg og læring træner tal og bogstaver.
*APPS*
👉 *Reklamelink* - https://track.adtraction.com/t/t?a=1646486406&as=1772625203&t=2&tk=1 - *SUPER GOD matematikapp* til de mindste. Gratis prøveperiode.
*LEKTIEHJÆLP*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=95261 - *LEKTIEHJÆLP* både til folkeskole og gymnasie niveau - *Prøv gratis 👩🎓*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=76792 - BOOST dine matematikevner med et matematikforløb eller lektiehjælp.
*LÆRINGSCAMP*
👉https://www.dincamp.dk/camps/matematikcamp/ - Tag på camp i din ferie: Et sjovt fagligt boost i matematik (eller andre fag)
#Matematik #Tælletræ #TælletræSandsynlighed #Folkeskolen #Lektier #Lektiehjælp #1klasse #2klasse #3klasse #4klasse #5klasse #6klasse #7klasse #RegnMedTælletræ #Tælletræ #LærLetMatematik #RegnTælletræ #RegnMedTælletræ #Skole #SkoleHjælp #UNIKSkole
Regn minus - Hvordan regner man minus let?
Her bruger vi matematik til at udregne minusstykker.
Se hvordan du opsætter et minus stykke så det er let at regne.
Folkeskolematematik
0. klasse matematik
1. Klasse matematik
2. Klasse matematik
3. Klasse matematik
4. Klasse matematik
*HJEMMESIDER OM MATEMATIK* 😃 (Video med en kort præsentation af de enkelte sider her: https://www.youtube.com/watch?v=nHfq43oEgxg )
👉 https://mattip.dk/ - Gratis opgaver og kopiark.
👉 https://matematikbogen.dk/ - Print let opgaver om plus, minus, gange og dividerer gratis.
👉 https://www.gratisskole.dk/ - Masser af gratis skolerelevante opgaver.
👉 https://www.legoglektie.dk/ - Hjemmeside med skolematerialer til børn fra 2-13 år. Gratis at bruge for private.
👉https://www.matematikbanken.dk/side/kompendier/ - Blandet opgaver målrettet 9-10 klasse.
👉https://www.opgaveskyen.dk/ - Opgaver til mange af folkeskolens fag. Klar til print.
👉https://undervisningsforlob.dk/matematik - Matematikopgaver og små spil klar til print. Målgruppe 5-12 år.
👉https://www.printland.dk/sjov-med-tal.aspx - Print opgaver til de helt små børn (børnehave og tidlig indskoling).
*BUTIKKER*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=69642 - Online-butik hvor man kan købe *piktogrammer,* læringsspil, opgaver, tavler, legetøj og læringscirkler. *Stort udvalg*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=64474&htmlurl=https://www.laerlet.dk/butik/ - Online-butik med materialer der via leg og læring træner tal og bogstaver.
*APPS*
👉 *Reklamelink* - https://track.adtraction.com/t/t?a=1646486406&as=1772625203&t=2&tk=1 - *SUPER GOD matematikapp* til de mindste. Gratis prøveperiode.
*LEKTIEHJÆLP*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=95261 - *LEKTIEHJÆLP* både til folkeskole og gymnasie niveau - *Prøv gratis 👩🎓*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=76792 - BOOST dine matematikevner med et matematikforløb eller lektiehjælp.
*LÆRINGSCAMP*
👉https://www.dincamp.dk/camps/matematikcamp/ - Tag på camp i din ferie: Et sjovt fagligt boost i matematik (eller andre fag)
#Matematik #Minus #Folkeskolen #Lektier #Lektiehjælp #0klasse #1klasse #2klasse #3klasse #4klasse #Minusstykker #UdregnMinus #LetMatematik #OpsætningAfMinus #UNIKSkole
Chancetræ - Hvad er det? Hvordan bruger man det til at regne?
Få svaret og en forklaring her hurtigt og let :)
Lær hvordan man bruger et chancetræ til at hjælpe med at regne med sandsynlighed. Videoen forklarer brugen af chancetræ og arbejder med forståelse samt eksempler.
Folkeskolematematik
1. Klasse matematik
2. Klasse matematik
3. Klasse matematik
4. Klasse matematik
5. Klasse matematik
6. Klasse matematik
7. Klasse matematik
*HJEMMESIDER OM MATEMATIK* 😃 (Video med en kort præsentation af de enkelte sider her: https://www.youtube.com/watch?v=nHfq43oEgxg )
👉 https://mattip.dk/ - Gratis opgaver og kopiark.
👉 https://matematikbogen.dk/ - Print let opgaver om plus, minus, gange og dividerer gratis.
👉 https://www.gratisskole.dk/ - Masser af gratis skolerelevante opgaver.
👉 https://www.legoglektie.dk/ - Hjemmeside med skolematerialer til børn fra 2-13 år. Gratis at bruge for private.
👉https://www.matematikbanken.dk/side/kompendier/ - Blandet opgaver målrettet 9-10 klasse.
👉https://www.opgaveskyen.dk/ - Opgaver til mange af folkeskolens fag. Klar til print.
👉https://undervisningsforlob.dk/matematik - Matematikopgaver og små spil klar til print. Målgruppe 5-12 år.
👉https://www.printland.dk/sjov-med-tal.aspx - Print opgaver til de helt små børn (børnehave og tidlig indskoling).
*BUTIKKER*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=69642 - Online-butik hvor man kan købe *piktogrammer,* læringsspil, opgaver, tavler, legetøj og læringscirkler. *Stort udvalg*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=64474&htmlurl=https://www.laerlet.dk/butik/ - Online-butik med materialer der via leg og læring træner tal og bogstaver.
*APPS*
👉 *Reklamelink* - https://track.adtraction.com/t/t?a=1646486406&as=1772625203&t=2&tk=1 - *SUPER GOD matematikapp* til de mindste. Gratis prøveperiode.
*LEKTIEHJÆLP*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=95261 - *LEKTIEHJÆLP* både til folkeskole og gymnasie niveau - *Prøv gratis 👩🎓*
👉 *Reklamelink* - https://www.partner-ads.com/dk/klikbanner.php?partnerid=46683&bannerid=76792 - BOOST dine matematikevner med et matematikforløb eller lektiehjælp.
*LÆRINGSCAMP*
👉https://www.dincamp.dk/camps/matematikcamp/ - Tag på camp i din ferie: Et sjovt fagligt boost i matematik (eller andre fag)
#Matematik #Chancetræ #ChancetræSandsynlighed #Folkeskolen #Lektier #Lektiehjælp #1klasse #2klasse #3klasse #4klasse #5klasse #6klasse #7klasse #RegnMedChance #Chance #LærLetMatematik #RegnChancetræ #RegnMedChancetræ #Skole #SkoleHjælp #UNIKSkole
Matematik er bare så let, hvis mestring af Mange får forrang for mestring af matematik. Et barn har allerede sin egne Mange-mestring som 3årig ”Hvor gammel bliver du næste gang?”. Svaret kommer straks med 4 fingre løftet i vejret. Men der protesteres kraftigt, hvis man viser fire fingre samlet to og to: ”Det er ikke fire, det er 2 toere.” Så barnet kan skelne mellem eksistens og essens. Det ser, hvad der eksisterer i rum og tid, bundter med to i rum, optalt til to i tid. Et barns tal-sprog bygger på 2-dimensionale bundt-tal med enheder, som skolen kunne bygge videre på i stedet for at påtvinge barnet 1-dimensionale linje-tal uden enheder, der bygger på den grundantagelse, at 2 + 1 er 3 altid, og ikke kun hvis enhederne er ens. Dette til trods for at 2par + 1 er 5. Denne selvskabte og selvrefererende ’matema-tisme’ uden enheder er således altid er sand indenfor, men sjældent uden for skolen. Og ved at lade essensen kvæle eksistensen, kvæler matematismen også barnets eget tal-sprog og medfødte talsans. Hvorved skolen i stedet for almen tal-kyndighed skaber udbredt faglig eksklusion og tal-blindhed , der senere åbner op for skandaler inden for både det personlige og sociale liv. En skandale som forvandles til et mirakel ved at skolen bruger barnets eget tal-sprog.
Program:
09 - 09.40
Brøk #1
- Brøk plus en brøk
- Brøk gange en brøk
Opgaver med plus brøker:
https://indd.adobe.com/view/83....c9afe3-30ba-4629-878
Opgaver med et tal gange en brøk:
https://indd.adobe.com/view/8e....7fc245-bcd7-4c03-9e3
10.15 - 11
Ligninger #1
- Introduktion til ligninger
- Ligninger med plus og et X
Hvordan forlænger man brøker, lær at forlænge brøker på 2 minutter. En nem og hurtig måde at forstår hvordan man forlænger brøker.
Gratis matematik opgaver:
https://indd.adobe.com/view/74....4601db-27c8-4964-85e
#matematikerlet
#hjælptilmatematik
#gratismatematikopgaver
#brøkregning
#brøker
Hvordan minusser man en brøk med en brøk.
2/3-1/3, hvordan gør man. Hvordan trækker man en brøk fra en brøk.
Gratis matematik opgaver til video'en med facit liste:
https://indd.adobe.com/view/e3....6669cc-9c0f-4194-a51
#matematikerlet
#hjælptilmatematik
#gratismatematikopgaver
#brøkregning
#brøker
Hvordan ganger man et heltal med en brøk:
Gratis matematik opgaver til video'en:
https://indd.adobe.com/view/8e....7fc245-bcd7-4c03-9e3
#matematikerlet
#hjælptilmatematik
#gratismatematikopgaver
#brøkregning
#brøker
En guide til hvordan du opnår den perfekt foldede skjorte. Vi fortæller dig hvordan du folder din skjorte, så den ligger flot og klar til brug. Vi har den holdning, at skjorter skal være i en skuffe eller på en hylde. De skal ikke masses ind i et overfyldt skab på en bøjle...hvor de risikerer at blive krøllet igen!
En foldet skjorte fylder mindre, skaber bedre overblik og er lige klar til brug eller smide i kufferten, når du skal på forretningsrejse.
God fornøjelse
Beskrivelse af hvordan man ganger med kommatal (for 4. klasse)
Du skal kunne indsætte tal i en formel med ubekendte.
Du skal kunne isolere forskellige ubekendte i en formel.
Du skal kunne løse et matematisk problem ved at opstille en ligning.
Original titel: "Dividing a whole number by a decimal"
https://www.khanacademy.org/ma....th/cc-fifth-grade-ma
Eksempel på at dividere et trecifret, helt tal med et decimaltal.
2 matematiknørder fra 5 kl. Viser division. På en meget nem måde
I denne video om brøker viser jeg hvordan man ganger og dividere brøker
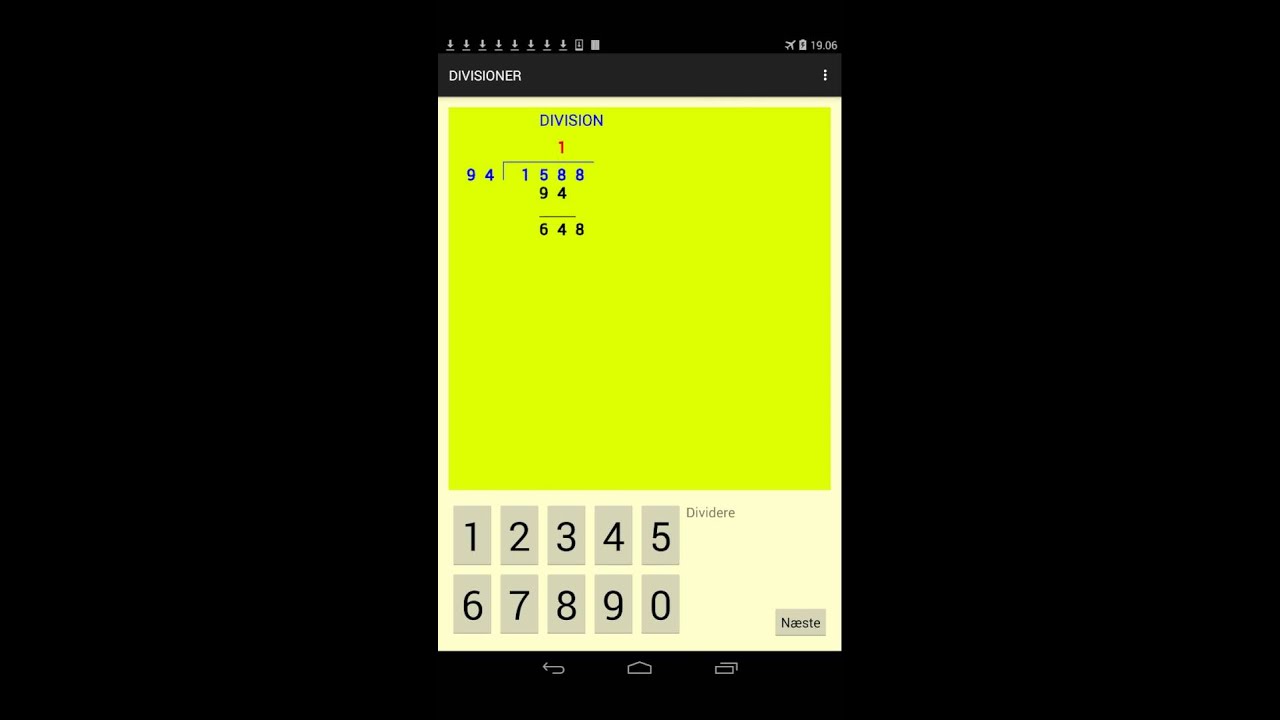
DIVISION
Du kan lære at dele tal sammensat af forskellige cifre ved hjælp af standard algoritme.
Du kan ændre antallet af cifre i tæller og nævner.
Du kan vælge mellem mulighederne, fortsætte eller genstarte, hvis du begår fejl.